CSE-250 Fall 2022 - Section B - Average Runtime, Stacks, Queues

Average Runtime, Stacks, Queues

CSE-250 Fall 2022 - Section B

Oct 3, 2022

Textbook: Ch. 7

Stacks

A stack of objects on top of one another.

Push: Put a new object on top of the stack
Pop: Remove the object on top of the stack
Top: Peek at what's on top of the stack

Queue

Outside of the US, "queueing" is lining up.

Enqueue: Put a new object at the end of the queue
Dequeue: Remove the next object in the queue
Head: Peek at the next object in the queue

Thought question: How could you use an array to build a queue?

ArrayBuffer Attempt 1

Enqueue: Append
Dequeue: Remove(0)

What's the complexity?

ArrayBuffer Attempt 2

Enqueue: Insert(0)
Dequeue: Remove(last)

What's the complexity?

Can we avoid having to move all of the
elements forward or backward a spot?

ArrayDequeue (Resizable Ring Buffer)

Active Array = [start, end)

Enqueue: Resize buffer if needed; Add new element at buffer[end]; Advance end pointer (wrap around to front)
Dequeue: Remove element at buffer[start]; Advance start pointer (wrap around to front)

What's the complexity?

Applications of Stacks and Queues

Stack: Checking for Balanced Parenthesis/Braces
Queue: Scheduling Packets for Delivery
Both: Searching Mazes

Balanced Parenthesis/Braces

What does it mean for parenthesis/braces to be balanced?

Every opening symbol is matched by a closing symbol
No nesting overlaps (e.g., {(}) is not ok).

`{()({})}`	`{())`	`())`
✅	❌	❌

Balanced Parenthesis/Braces

Idea: Count the number of unmatched open parens, braces.

Increment counter on (, decrement on )

Problem: allows {(})

Balanced Parenthesis/Braces

Idea: Track nesting on a stack!

On ( or {, push the symbol on the stack.

On ) or }, pop the stack and check the popped symbol.

Network Packets

Router: 1gb/s internal network, 100mb/s external

1gb/s sent to router, but only 100mb/s can leave.
How do packets get delivered?

Queues

Enqueue data packets in the order they are received.
When outgoing bandwidth available, dequeue and send.

Avoiding Queueing Delays

Limit size of queue; Packets that don't fit are dropped

TCP: blocked packets are retried UDP: application deals with dropped packets.

Mazes

O is the start, X is the objective
- There may be multiple paths
- Generally, we want the shortest
Approach 1: Take the first available route in one direction
- Right, Down, Left, or Up
- Down, Right, Up, Left

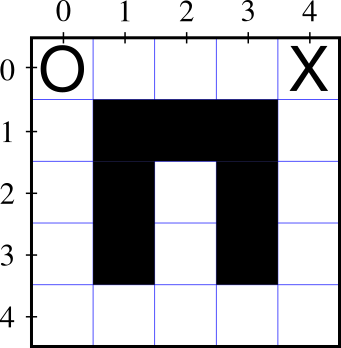
Mazes

O is the start, X is the objective
- There may be multiple paths
- Generally, we want the shortest
Approach 1: Take the first available route in one direction
- Right, Down, Left, or Up
- Down, Right, Up, Left

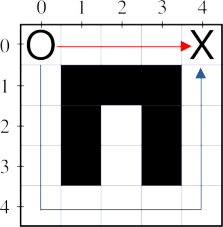
Mazes

How do you know which one is best?

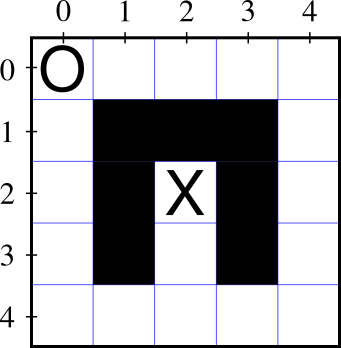
Mazes

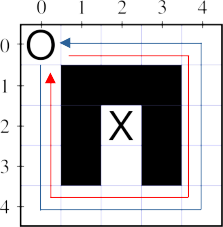
Priority order doesn't guarantee exploring the entire maze

Formalizing Maze-Solving

Inputs:

The map: An $n\times m$ grid of filled/empty squares.
The O is at position $start$
The X is at position $dest$

Goal:

Compute $steps(\texttt{start}, \texttt{dest})$, the minimum steps from start to end.

How do we define $steps$?

Formalizing Maze-Solving

$$steps(\texttt{pos},\texttt{dest}) = \begin{cases} 0 & \textbf{if } \texttt{pos} = \texttt{dest}\\ \end{cases}$$

Formalizing Maze-Solving

$$steps(\texttt{pos},\texttt{dest}) = \begin{cases} 0 & \textbf{if } \texttt{pos} = \texttt{dest}\\ \infty & \textbf{if } is\_filled(\texttt{pos})\\ \end{cases}$$

Formalizing Maze-Solving

$$steps(\texttt{pos},\texttt{dest}) = \begin{cases} 0 & \textbf{if } \texttt{pos} = \texttt{dest}\\ \infty & \textbf{if } is\_filled(\texttt{pos})\\ 1 + min\_adjacent(\texttt{pos}, \texttt{dest}) & \textbf{otherwise} \end{cases}$$

where...

$min\_adjacent(\texttt{pos}, \texttt{dest}) = $ $$\min\begin{cases} steps(moveRight(\texttt{pos}), \texttt{dest})\\ steps(moveDown(\texttt{pos}), \texttt{dest})\\ steps(moveLeft(\texttt{pos}), \texttt{dest})\\ steps(moveUp(\texttt{pos}), \texttt{dest})\\ \end{cases}$$

$steps(\texttt{pos}, \texttt{dest})$

if pos == dest then return 0
elif is_filled(pos) then return ∞
else return 1 + min of
- $steps(moveRight(\texttt{pos}, \texttt{dest}))$
- $steps(moveDown(\texttt{pos}, \texttt{dest}))$
- $steps(moveLeft(\texttt{pos}, \texttt{dest}))$
- $steps(moveUp(\texttt{pos}, \texttt{dest}))$

Problem: Infinite loop!

Insight: A path with a loop in it can't
be shorter than one without the loop.

Mark nodes as visited

$steps(\texttt{pos}, \texttt{dest})$

if pos == dest then return 0
elif pos marked visited then return ∞
elif is_filled(pos) then return ∞
else
- Mark pos as visited
- return 1 + min of all 4 steps

Problem: The first time you visit a node,
it may be via a longer path!

Unmark nodes as you leave them

$steps(\texttt{pos}, \texttt{dest})$

if pos == dest then return 0
elif pos marked visited then return ∞
elif is_filled(pos) then return ∞
else
- Mark pos as visited
- stepCount = 1 + min of all 4 steps
- Unmark pos as visited
- return stepCount

Maze-Solving

Inputs:

The map: An $n\times m$ grid of filled/empty squares.
The O is at position $start$
The X is at position $dest$

Goal:

Compute $steps(\texttt{start}, \texttt{dest})$, the minimum steps from start to end.

What path did we take?

Idea:

Follow the nodes marked visited

$steps(\texttt{pos}, \texttt{dest}, \texttt{visited})$

if pos == dest then return visited.copy()
elif pos ∈ visited return no_path
elif is_filled(pos) then return no_path
else
- visited.append(pos)
- bestPath = min of all 4 one-step paths
- visited.removeLast()
- return bestPath

$steps(\texttt{pos}, \texttt{dest}, \texttt{visited})$

if pos == dest then return visited.copy()
elif pos ∈ visited return no_path
elif is_filled(pos) then return no_path
else
- visited.push(pos)
- bestPath = min of all 4 one-step paths
- visited.pop()
- return bestPath

Thought question: Can you solve a maze
with a queue instead?